Hang-Hyun Jo , Eun Lee, and Young-Ho Eom
Physical Review E 104.5 (2021): 054301.
Abstract
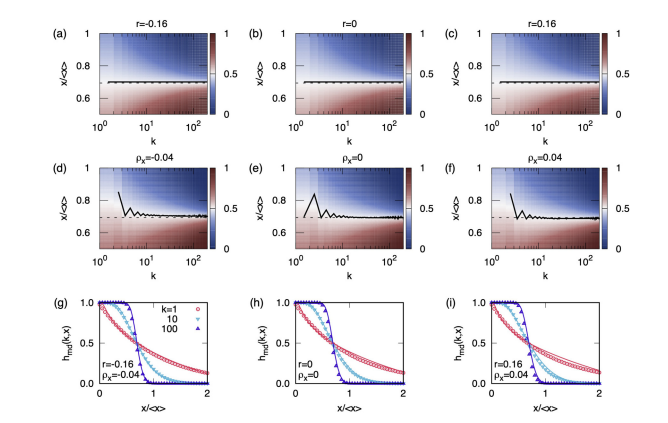
One of the interesting phenomena due to the topological heterogeneities in complex networks is the friendship paradox, stating that your friends have on average more friends than you do. Recently, this paradox has been generalized for arbitrary nodal attributes, called a generalized friendship paradox (GFP). In this paper, we analyze the GFP for the networks in which the attributes of neighboring nodes are correlated with each other. The correlation structure between attributes of neighboring nodes is modeled by the Farlie-Gumbel-Morgenstern copula, enabling us to derive approximate analytical solutions of the GFP for three kinds of methods summarizing the neighborhood of the focal node, i.e., mean-based, median-based, and fraction-based methods. The analytical solutions are comparable to simulation results, while some systematic deviations between them might be attributed to the higher-order correlations between nodal attributes. These results help us get deeper insight into how various summarization methods as well as the correlation structure of nodal attributes affect the GFP behavior, hence better understand various related phenomena in complex networks.