Dongsu Bak ,a Chanju Kim b and Sang-Heon Yi c
aPhysics Department & Natural Science Research Institute,
University of Seoul, Seoul 02504, Korea
bDepartment of Physics, Ewha Womans University,
Seoul 03760, Korea
cCenter for Quantum Spacetime & Physics Department,
Sogang University, Seoul 04107, Korea
E-mail: dsbak@uos.ac.kr, cjkim@ewha.ac.kr, shyi@sogang.ac.kr
Abstract: We consider a time-dependent O(1/G) deformation of pure de Sitter (dS) space
in dS gravity coupled to a massless scalar field. It is the dS counterpart of the AdS Janus
deformation and interpolates two asymptotically dS spaces in the far past and the far future
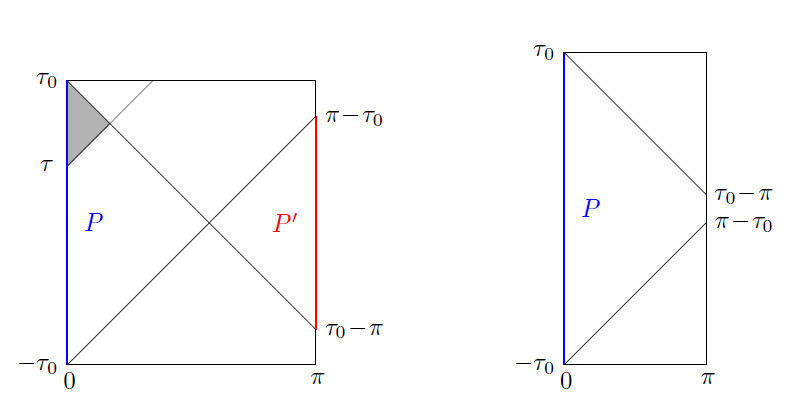
with a single deformation parameter. The Penrose diagram can be elongated along the
time direction indefinitely as the deformation becomes large. After studying the classical
properties of the geometry such as the area theorem and the fluctuation by a matter field,
we explore the algebraic structure of the field operators on the deformed spacetime. We
argue that the algebra is a von Neumann factor of type II∞ for small deformations, but
there occurs a transition to type I∞ as the deformation increases so that the neck region
of the deformed space becomes a Lorentzian cylinder.
Keywords: Classical Theories of Gravity, de Sitter space, Models of Quantum Gravity,
Space-Time Symmetries
ArXiv ePrint: 2407.04316
Open